Mathematical Information Studies 9

| Lecturer | Takafumi KANAMORI, Associate Professor |
|---|---|
| Department | School of Informatics and Sciences, 2011 Fall |
| Recommended for: | School of Informatics and Science (2・One session / week 15 weeks / semester) |
Key Features
Lecture notes and assignments are uploaded on the web-site. While the main focus of the course is to introduce the mathematical basis of statistics, some examples using real-world data will also be shown.
Course Objectives
To understand the mathematical foundations of statistics and various applications of data analysis.
Course Requirements and Recommended Courses
Prior basic knowledge of analytics, linear algebra and probability is recommended.
Textbook
No textbooks are assigned.
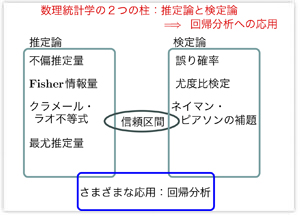
Course Schedule
| Session | Contents |
|---|---|
| 1 | Guidance |
| 2 | Elements of probability I |
| 3 | Elements of probability II |
| 4 | Statistical inference I (unbiased estimator) |
| 5 | Statistical inference II (Fisher information) |
| 6 | Statistical inference III (Cramer-Rao inequality) |
| 7 | Confidence interval I (estimation with confidence) |
| 8 | Confidence interval II (confidence interval and the t-distribution) |
| 9 | Hypothesis Testing I (null hypothesis and alternative hypothesis) |
| 10 | Hypothesis testing II (errors in statistical test) |
| 11 | Hypothesis testing III (optimality, Neyman-Pearson lemma) |
| 12 | Linear regression I (inference of regression function) |
| 13 | Linear regression II (confidence interval and statistical test in linear regression) |
| 14 | Maximum likelihood estimator I (maximum likelihood estimator) |
| 15 | Maximum likelihood estimator II (exponential family) |
Grading
Grading will be based on homework assignments.
Last updated
January 14, 2020
